- Research Article
- Open access
- Published:
On Some Arithmetical Properties of the Genocchi Numbers and Polynomials
Advances in Difference Equations volume 2008, Article number: 195049 (2009)
Abstract
We investigate the properties of the Genocchi functions and the Genocchi polynomials. We obtain the Fourier transform on the Genocchi function. We have the generating function of  -Genocchi polynomials. We define the Cangul-Ozden-Simsek's type twisted
-Genocchi polynomials. We define the Cangul-Ozden-Simsek's type twisted  -Genocchi polynomials and numbers. We also have the generalized twisted
-Genocchi polynomials and numbers. We also have the generalized twisted  -Genocchi numbers attached to the Dirichlet's character
-Genocchi numbers attached to the Dirichlet's character  . Finally, we define zeta functions related to
. Finally, we define zeta functions related to  -Genocchi polynomials and have the generating function of the generalized
-Genocchi polynomials and have the generating function of the generalized  -Genocchi numbers attached to
-Genocchi numbers attached to  .
.
1. Introduction
After Carlitz introduced an interesting  -analogue of Frobenius-Euler numbers in [1],
-analogue of Frobenius-Euler numbers in [1],  -Bernoulli and
-Bernoulli and  -Euler numbers and polynomials have been studied by several authors. Recently, many authors have an interest in the
-Euler numbers and polynomials have been studied by several authors. Recently, many authors have an interest in the  -extension of the Genocchi numbers and polynomials(cf. [2–5]). Kim et al. [5] defined the
-extension of the Genocchi numbers and polynomials(cf. [2–5]). Kim et al. [5] defined the  -Genocchi numbers and the
-Genocchi numbers and the  -Genocchi polynomials. In [3], Kim derived the
-Genocchi polynomials. In [3], Kim derived the  -analogs of the Genocchi numbers and polynomials by constructing
-analogs of the Genocchi numbers and polynomials by constructing  -Euler numbers. He also gave some interesting relations between
-Euler numbers. He also gave some interesting relations between  -Euler and
-Euler and  -Genocchi numbers. The first author et al. [6] obtained the distribution relation for the Genocchi polynomials.
-Genocchi numbers. The first author et al. [6] obtained the distribution relation for the Genocchi polynomials.
The main aim of this paper is to derive the Fourier transform for the Genocchi function. Recently, Kim [7] investigated the properties of the Euler functions and derived the interesting formula related to the infinite series by using the Fourier transform for the Euler function. In this paper, we investigate some arithmetical properties of the Genocchi functions and the Genocchi polynomials.
In [8], Cangul-Ozden-Simsek constructed new generating functions of the twisted  -extension of twisted Euler polynomials and numbers attached to the Dirichlet character
-extension of twisted Euler polynomials and numbers attached to the Dirichlet character  . Cangul et al. [8] also defined the twisted
. Cangul et al. [8] also defined the twisted  -extension of zeta functions, which interpolate the twisted
-extension of zeta functions, which interpolate the twisted  -extension of Euler numbers at negative integers. In this paper, we define the Cangul-Ozden-Simsek type twisted
-extension of Euler numbers at negative integers. In this paper, we define the Cangul-Ozden-Simsek type twisted  -Genocchi polynomials and numbers. We have the generating function of
-Genocchi polynomials and numbers. We have the generating function of  -Genocchi polynomials. We have the generalized twisted
-Genocchi polynomials. We have the generalized twisted  -Genocchi numbers attached to the Dirichlet character
-Genocchi numbers attached to the Dirichlet character  . We define zeta functions related to
. We define zeta functions related to  -Genocchi polynomials and we have the generating function of the generalized
-Genocchi polynomials and we have the generating function of the generalized  -Genocchi numbers attached to
-Genocchi numbers attached to  .
.
Let  be a fixed odd prime number. Throughout this paper
be a fixed odd prime number. Throughout this paper  ,
,  , and
, and  will, respectively, denote the ring of
will, respectively, denote the ring of  -adic rational integers, the field of
-adic rational integers, the field of  -adic rational numbers, the complex number field, and the completion of algebraic closure of
-adic rational numbers, the complex number field, and the completion of algebraic closure of  . Let
. Let  be the set of natural numbers and
be the set of natural numbers and  . Let
. Let  be the normalized exponential valuation of
be the normalized exponential valuation of  with
with  When one talks of
When one talks of  -extension,
-extension,  is variously considered as an indeterminate, a complex number
is variously considered as an indeterminate, a complex number  or a
or a  -adic number
-adic number  . If
. If  , one normally assumes
, one normally assumes  If
If  , then we assume
, then we assume  . We also use the following notations:
. We also use the following notations:

For  , a fixed positive integer with
, a fixed positive integer with  , set
, set

where  satisfies the condition
satisfies the condition  . The distribution is defined by
. The distribution is defined by

We say that  is uniformly differential function at a point
is uniformly differential function at a point  , and we write
, and we write  , if the difference quotients,
, if the difference quotients,  have a limit
have a limit  as
as  .
.
For  , the
, the  -adic invariant
-adic invariant  -integral on
-integral on  is defined as
is defined as

The fermionic  -adic
-adic  -measures on
-measures on  are defined as
are defined as

and the fermionic  -adic invariant
-adic invariant  -integral on
-integral on  is defined as
is defined as

for  . For
. For  , we note that
, we note that

where  . (For details see [1–44].)
. (For details see [1–44].)
In this paper, we investigate arithmetical properties of the Genocchi functions and the Genocchi polynomials. In Section 2, we derive the Fourier transform on the Genocchi function. In Section 3, we define the Cangul-Ozden-Simsek type twisted  -Genocchi polynomials and numbers. We have the generating function of
-Genocchi polynomials and numbers. We have the generating function of  -Genocchi polynomials. We also have the generalized twisted
-Genocchi polynomials. We also have the generalized twisted  -Genocchi numbers attached to
-Genocchi numbers attached to  . In Section 4, we define zeta functions related to
. In Section 4, we define zeta functions related to  -Genocchi polynomials and we have the generating function of the generalized
-Genocchi polynomials and we have the generating function of the generalized  -Genocchi numbers attached to
-Genocchi numbers attached to  .
.
2. Genocchi Numbers and Functions
The Genocchi numbers are defined as

where we use the technique method notation by replacing  by
by  , symbolically. From this definition, we can derive the following relation:
, symbolically. From this definition, we can derive the following relation:

From (2.2), we note that  ,
,  ,
,  and
and  . The Genocchi polynomials
. The Genocchi polynomials  are defined as
are defined as

From (2.1) and (2.3), we can derive

By (2.1), it is not difficult to show that the recurrence relation for the Genocchi numbers is given by

where  is the Kronecker symbol.
is the Kronecker symbol.
From (2.4) and (2.5), we note that

Thus, we obtain the following lemma.
Lemma 2.1.
For
 , one has
, one has
 .
.
From (2.4), we can easily derive

By (2.7), we obtain the following proposition.
Proposition 2.2.
For
 , one has
, one has

From now on, we assume that  is the Genocchi function. Let us consider the Fourier transform for the Genocchi function
is the Genocchi function. Let us consider the Fourier transform for the Genocchi function  as follows.
as follows.
For  , the Fourier transform on the Genocchi function is given by
, the Fourier transform on the Genocchi function is given by

where

From (2.8) and (2.10), we note that

Thus, for  , we have
, we have

From (2.4) and (2.10), we derive

From (2.12) and (2.13), we can derive

By (2.9) and (2.14), we have that  and
and

Therefore, we obtain the following theorem.
Theorem 2.3.
For
 with
with
 , one has
, one has

If we take  , then we have
, then we have

By (2.17) and Lemma 2.1, we obtain the following corollary.
Corollary 2.4.
For
 , one has
, one has

From Corollary 2.4, we note that

Thus, we have

By (2.20), we obtain the following corollary.
Corollary 2.5.
For
 , one has
, one has

3. 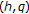 -Extension of Twisted Genocchi Numbers and Polynomials
-Extension of Twisted Genocchi Numbers and Polynomials
In this section, we will define the  -extensions of twisted Genocchi numbers and polynomials which are the Cangul-Ozden-Simsek type twisted
-extensions of twisted Genocchi numbers and polynomials which are the Cangul-Ozden-Simsek type twisted  -Genocchi numbers and polynomials, respectively. We will have the generating function of
-Genocchi numbers and polynomials, respectively. We will have the generating function of  -Genocchi polynomials and the generalized twisted
-Genocchi polynomials and the generalized twisted  -Genocchi numbers attached to
-Genocchi numbers attached to  .
.
Let  . Then, we have from the definition of the Genocchi numbers and the fermionic
. Then, we have from the definition of the Genocchi numbers and the fermionic  -adic
-adic  -integral on
-integral on  that
that

Thus, we obtain

For  and
and  , we have
, we have

By (3.2) and (3.3), if we take  , we easily see that
, we easily see that

Thus, we have

If  , then we know that
, then we know that

Thus, we get

We can consider the generalized Genocchi numbers as follows:

where  . Let
. Let  with
with  . From (3.3) and (3.8), we note that
. From (3.3) and (3.8), we note that

By (3.8) and (3.9), it is not difficult to show that

Thus, the distribution relations for the Genocchi numbers and the Genocchi polynomials for  with
with  are obtained as follows (cf. [6]):
are obtained as follows (cf. [6]):

By using the multivariate integral, we can also consider the multiple Genocchi numbers and polynomials.
Let  with
with  be indeterminate and let
be indeterminate and let  . Then, we note that
. Then, we note that

Now, we define the Cangul-Ozden-Simsek type  -Genocchi polynomials
-Genocchi polynomials  as follows:
as follows:

From (3.13), we note that

Let  be the space of primitive
be the space of primitive  -th root of unity with
-th root of unity with

and let  be the direct limit of
be the direct limit of  , that is,
, that is,

and then  is a
is a  -adic locally constant space.
-adic locally constant space.
For  , we define the Cangul-Ozden-Simsek type twisted
, we define the Cangul-Ozden-Simsek type twisted  -Genocchi polynomials
-Genocchi polynomials  as follows:
as follows:

By (3.17), we have

From the result of Cangul et al. [8], we note that

where  is the twisted
is the twisted  -Euler polynomials.
-Euler polynomials.
Let  be the Dirichlet character with conductor
be the Dirichlet character with conductor  with
with  . Then, we consider the generalized Genocchi numbers attached to
. Then, we consider the generalized Genocchi numbers attached to  as follows:
as follows:

where  .
.
From (3.3) and (3.20), we note that

By (3.20) and (3.21), it is not difficult to show that

Now, we also consider the Cangul-Ozden-Simsek type twisted  -Genocchi numbers attached to
-Genocchi numbers attached to  as follows.
as follows.
For  and
and  , we have
, we have

From (3.23), we have

From the result of Cangul et al. [8], we note that

where  are called the generalized twisted
are called the generalized twisted  -Euler numbers attached to
-Euler numbers attached to  .
.
4. Zeta Functions Related to the Genocchi Polynomials
In this section, we assume that  with
with  . Let
. Let  be the generating function of
be the generating function of  -Genocchi polynomials defined as follows:
-Genocchi polynomials defined as follows:

where  .
.
Then, we note that

By (4.1) and (4.2), we easily see that

for  . Therefore, we obtain the following proposition.
. Therefore, we obtain the following proposition.
Proposition 4.1.
For
 , one has
, one has

From Proposition 4.1, we can derive the Genocchi zeta function which interpolates Genocchi polynomials related to  -Genocchi polynomials at negative integers.
-Genocchi polynomials at negative integers.
For  , we define the Hurwitz-type Genocchi zeta functions related to
, we define the Hurwitz-type Genocchi zeta functions related to  -Genocchi polynomials and numbers as follows.
-Genocchi polynomials and numbers as follows.
Definition 4.2.
For
 , one has
, one has

By Proposition 4.1 and Definition 4.2, we obtain the following theorem.
Theorem 4.3.
For
 , one has
, one has

The generating function of the generalized  -Genocchi numbers attached to
-Genocchi numbers attached to  is given by
is given by

where  ,
,  with
with  . Therefore, we have
. Therefore, we have

where  is a nontrivial character with conductor
is a nontrivial character with conductor  with
with  . From (4.8), it follows that
. From (4.8), it follows that

This is equivalent to

For  with
with  , let
, let  be a primitive Dirichlet character with conductor
be a primitive Dirichlet character with conductor  . Then, we define
. Then, we define

From (4.10) and (4.11), we obtain the following theorem.
Theorem 4.4.
For
 , one has
, one has

References
Carlitz L:
 -Bernoulli numbers and polynomials. Duke Mathematical Journal 1948, 15(4):987-1000. 10.1215/S0012-7094-48-01588-9
-Bernoulli numbers and polynomials. Duke Mathematical Journal 1948, 15(4):987-1000. 10.1215/S0012-7094-48-01588-9Kim T: Sums of powers of consecutive
 -integers. Advanced Studies in Contemporary Mathematics 2004, 9(1):15-18.
-integers. Advanced Studies in Contemporary Mathematics 2004, 9(1):15-18.Kim T: On the
 -extension of Euler and Genocchi numbers. Journal of Mathematical Analysis and Applications 2007, 326(2):1458-1465. 10.1016/j.jmaa.2006.03.037
-extension of Euler and Genocchi numbers. Journal of Mathematical Analysis and Applications 2007, 326(2):1458-1465. 10.1016/j.jmaa.2006.03.037Kim T: Note on
 -Genocchi numbers and polynomials. Advanced Studies in Contemporary Mathematics 2008, 17(1):9-15.
-Genocchi numbers and polynomials. Advanced Studies in Contemporary Mathematics 2008, 17(1):9-15.Kim T, Jang L-C, Pak HK: A note on
 -Euler and Genocchi numbers. Proceedings of the Japan Academy. Series A 2001, 77(8):139-141. 10.3792/pjaa.77.139
-Euler and Genocchi numbers. Proceedings of the Japan Academy. Series A 2001, 77(8):139-141. 10.3792/pjaa.77.139Rim S-H, Park KH, Moon EJ: On Genocchi numbers and polynomials. Abstract and Applied Analysis 2008, 2008:-7.
Kim T: Note on the Euler numbers and polynomials. Advanced Studies in Contemporary Mathematics 2008, 17(2):131-136.
Cangul IN, Ozden H, Simsek Y: Generating functions of the
 extension of twisted Euler polynomials and numbers. Acta Mathematica Hungarica 2008, 120(3):281-299. 10.1007/s10474-008-7139-1
extension of twisted Euler polynomials and numbers. Acta Mathematica Hungarica 2008, 120(3):281-299. 10.1007/s10474-008-7139-1Carlitz L:
 -Bernoulli and Eulerian numbers. Transactions of the American Mathematical Society 1954, 76: 332-350.
-Bernoulli and Eulerian numbers. Transactions of the American Mathematical Society 1954, 76: 332-350.Cenkci M: The
 -adic generalized twisted
-adic generalized twisted  -Euler-
-Euler- -function and its applications. Advanced Studies in Contemporary Mathematics 2007, 15(1):37-47.
-function and its applications. Advanced Studies in Contemporary Mathematics 2007, 15(1):37-47.Cenkci M, Can M: Some results on
 -analogue of the Lerch zeta function. Advanced Studies in Contemporary Mathematics 2006, 12(2):213-223.
-analogue of the Lerch zeta function. Advanced Studies in Contemporary Mathematics 2006, 12(2):213-223.Cenkci M, Can M, Kurt V:
 -adic interpolation functions and Kummer-type congruences for
-adic interpolation functions and Kummer-type congruences for  -twisted and
-twisted and  -generalized twisted Euler numbers. Advanced Studies in Contemporary Mathematics 2004, 9(2):203-216.
-generalized twisted Euler numbers. Advanced Studies in Contemporary Mathematics 2004, 9(2):203-216.Kim T: On
 -adic
-adic  -
- -functions and sums of powers. Discrete Mathematics 2002, 252(1–3):179-187.
-functions and sums of powers. Discrete Mathematics 2002, 252(1–3):179-187.Kim T:
 -Volkenborn integration. Russian Journal of Mathematical Physics 2002, 9(3):288-299.
-Volkenborn integration. Russian Journal of Mathematical Physics 2002, 9(3):288-299.Kim T: On Euler-Barnes multiple zeta functions. Russian Journal of Mathematical Physics 2003, 10(3):261-267.
Kim T: Analytic continuation of multiple
 -zeta functions and their values at negative integers. Russian Journal of Mathematical Physics 2004, 11(1):71-76.
-zeta functions and their values at negative integers. Russian Journal of Mathematical Physics 2004, 11(1):71-76.Kim T:
 -Riemann zeta function. International Journal of Mathematics and Mathematical Sciences 2004, 2004(12):599-605. 10.1155/S0161171204307180
-Riemann zeta function. International Journal of Mathematics and Mathematical Sciences 2004, 2004(12):599-605. 10.1155/S0161171204307180Kim T: Power series and asymptotic series associated with the
 -analog of the two-variable
-analog of the two-variable  -adic
-adic  -function. Russian Journal of Mathematical Physics 2005, 12(2):186-196.
-function. Russian Journal of Mathematical Physics 2005, 12(2):186-196.Kim T: A new approach to
 -adic
-adic  -
- -functions. Advanced Studies in Contemporary Mathematics 2006, 12(1):61-72.
-functions. Advanced Studies in Contemporary Mathematics 2006, 12(1):61-72.Kim T: Multiple
 -adic
-adic  -function. Russian Journal of Mathematical Physics 2006, 13(2):151-157. 10.1134/S1061920806020038
-function. Russian Journal of Mathematical Physics 2006, 13(2):151-157. 10.1134/S1061920806020038Kim T:
 -extension of the Euler formula and trigonometric functions. Russian Journal of Mathematical Physics 2007, 14(3):275-278. 10.1134/S1061920807030041
-extension of the Euler formula and trigonometric functions. Russian Journal of Mathematical Physics 2007, 14(3):275-278. 10.1134/S1061920807030041Kim T: On
 -adic interpolating function for
-adic interpolating function for  -Euler numbers and its derivatives. Journal of Mathematical Analysis and Applications 2008, 339(1):598-608. 10.1016/j.jmaa.2007.07.027
-Euler numbers and its derivatives. Journal of Mathematical Analysis and Applications 2008, 339(1):598-608. 10.1016/j.jmaa.2007.07.027Kim T:
 -Bernoulli numbers and polynomials associated with Gaussian binomial coefficients. Russian Journal of Mathematical Physics 2008, 15(1):51-57.
-Bernoulli numbers and polynomials associated with Gaussian binomial coefficients. Russian Journal of Mathematical Physics 2008, 15(1):51-57.Kim T: The modified
 -Euler numbers and polynomials. Advanced Studies in Contemporary Mathematics 2008, 16(2):161-170.
-Euler numbers and polynomials. Advanced Studies in Contemporary Mathematics 2008, 16(2):161-170.Kim T: On the symmetries of the
 -Bernoulli polynomials. Abstract and Applied Analysis 2008, 2008:-7.
-Bernoulli polynomials. Abstract and Applied Analysis 2008, 2008:-7.Kim T: Symmetry
 -adic invariant integral on
-adic invariant integral on  for Bernoulli and Euler polynomials. Journal of Difference Equations and Applications 2008, 14(12):1267-1277. 10.1080/10236190801943220
for Bernoulli and Euler polynomials. Journal of Difference Equations and Applications 2008, 14(12):1267-1277. 10.1080/10236190801943220Kim T: Euler numbers and polynomials associated with zeta functions. Abstract and Applied Analysis 2008, 2008:-11.
Kim T: A note on some formulae for the
 -Euler numbers and polynomials. Proceedings of the Jangjeon Mathematical Society 2006, 9(2):227-232.
-Euler numbers and polynomials. Proceedings of the Jangjeon Mathematical Society 2006, 9(2):227-232.Kim T: A note on
 -Volkenborn integration. Proceedings of the Jangjeon Mathematical Society 2005, 8(1):13-17.
-Volkenborn integration. Proceedings of the Jangjeon Mathematical Society 2005, 8(1):13-17.Kim T: On the Sehee integral representation associated with
 -Riemann zeta function. Proceedings of the Jangjeon Mathematical Society 2004, 7(2):125-127.
-Riemann zeta function. Proceedings of the Jangjeon Mathematical Society 2004, 7(2):125-127.Kim T, Rim S-H, Simsek Y: A note on the alternating sums of powers of consecutive
 -integers. Advanced Studies in Contemporary Mathematics 2006, 13(2):159-164.
-integers. Advanced Studies in Contemporary Mathematics 2006, 13(2):159-164.Kim T, Simsek Y: Analytic continuation of the multiple Daehee
 -
- -functions associated with Daehee numbers. Russian Journal of Mathematical Physics 2008, 15(1):58-65.
-functions associated with Daehee numbers. Russian Journal of Mathematical Physics 2008, 15(1):58-65.Kim Y-H, Kim W, Jang L-C: On the
 -extension of Apostol-Euler numbers and polynomials. Abstract and Applied Analysis 2008, 2008:-10.
-extension of Apostol-Euler numbers and polynomials. Abstract and Applied Analysis 2008, 2008:-10.Jang L-C: Multiple twisted
 -Euler numbers and polynomials associated with
-Euler numbers and polynomials associated with  -adic
-adic  -integrals. Advances in Difference Equations 2008, 2008:-11.
-integrals. Advances in Difference Equations 2008, 2008:-11.Ozden H, Cangul IN, Simsek Y: Multivariate interpolation functions of higher-order
 -Euler numbers and their applications. Abstract and Applied Analysis 2008, 2008:-16.
-Euler numbers and their applications. Abstract and Applied Analysis 2008, 2008:-16.Ozden H, Simsek Y: A new extension of
 -Euler numbers and polynomials related to their interpolation functions. Applied Mathematics Letters 2008, 21(9):934-939. 10.1016/j.aml.2007.10.005
-Euler numbers and polynomials related to their interpolation functions. Applied Mathematics Letters 2008, 21(9):934-939. 10.1016/j.aml.2007.10.005Ozden H, Simsek Y, Cangul IN: Euler polynomials associated with
 -adic
-adic  -Euler measure. General Mathematics 2007, 15(2):24-37.
-Euler measure. General Mathematics 2007, 15(2):24-37.Ozden H, Simsek Y, Rim S-H, Cangul IN: A note on
 -adic
-adic  -Euler measure. Advanced Studies in Contemporary Mathematics 2007, 14(2):233-239.
-Euler measure. Advanced Studies in Contemporary Mathematics 2007, 14(2):233-239.Ryoo CS, Song H, Agarwal RP: On the roots of the
 -analogue of Euler-Barnes' polynomials. Advanced Studies in Contemporary Mathematics 2004, 9(2):153-163.
-analogue of Euler-Barnes' polynomials. Advanced Studies in Contemporary Mathematics 2004, 9(2):153-163.Simsek Y: Theorems on twisted
 -function and twisted Bernoulli numbers. Advanced Studies in Contemporary Mathematics 2005, 11(2):205-218.
-function and twisted Bernoulli numbers. Advanced Studies in Contemporary Mathematics 2005, 11(2):205-218.Simsek Y: On
 -adic twisted
-adic twisted  -
- -functions related to generalized twisted Bernoulli numbers. Russian Journal of Mathematical Physics 2006, 13(3):340-348. 10.1134/S1061920806030095
-functions related to generalized twisted Bernoulli numbers. Russian Journal of Mathematical Physics 2006, 13(3):340-348. 10.1134/S1061920806030095Simsek Y: Generating functions of the twisted Bernoulli numbers and polynomials associated with their interpolation functions. Advanced Studies in Contemporary Mathematics 2008, 16(2):251-278.
Simsek Y, Yurekli O, Kurt V: On interpolation functions of the twisted generalized Frobenius-Euler numbers. Advanced Studies in Contemporary Mathematics 2007, 15(2):187-194.
Srivastava HM, Kim T, Simsek Y:
 -Bernoulli numbers and polynomials associated with multiple
-Bernoulli numbers and polynomials associated with multiple  -zeta functions and basic
-zeta functions and basic  -series. Russian Journal of Mathematical Physics 2005, 12(2):241-268.
-series. Russian Journal of Mathematical Physics 2005, 12(2):241-268.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Park, K.H., Kim, YH. On Some Arithmetical Properties of the Genocchi Numbers and Polynomials. Adv Differ Equ 2008, 195049 (2009). https://doi.org/10.1155/2008/195049
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1155/2008/195049
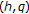 -Extension of Twisted Genocchi Numbers and Polynomials
-Extension of Twisted Genocchi Numbers and Polynomials -Bernoulli numbers and polynomials. Duke Mathematical Journal 1948, 15(4):987-1000. 10.1215/S0012-7094-48-01588-9
-Bernoulli numbers and polynomials. Duke Mathematical Journal 1948, 15(4):987-1000. 10.1215/S0012-7094-48-01588-9 -integers. Advanced Studies in Contemporary Mathematics 2004, 9(1):15-18.
-integers. Advanced Studies in Contemporary Mathematics 2004, 9(1):15-18. -extension of Euler and Genocchi numbers. Journal of Mathematical Analysis and Applications 2007, 326(2):1458-1465. 10.1016/j.jmaa.2006.03.037
-extension of Euler and Genocchi numbers. Journal of Mathematical Analysis and Applications 2007, 326(2):1458-1465. 10.1016/j.jmaa.2006.03.037 -Genocchi numbers and polynomials. Advanced Studies in Contemporary Mathematics 2008, 17(1):9-15.
-Genocchi numbers and polynomials. Advanced Studies in Contemporary Mathematics 2008, 17(1):9-15. -Euler and Genocchi numbers. Proceedings of the Japan Academy. Series A 2001, 77(8):139-141. 10.3792/pjaa.77.139
-Euler and Genocchi numbers. Proceedings of the Japan Academy. Series A 2001, 77(8):139-141. 10.3792/pjaa.77.139 extension of twisted Euler polynomials and numbers. Acta Mathematica Hungarica 2008, 120(3):281-299. 10.1007/s10474-008-7139-1
extension of twisted Euler polynomials and numbers. Acta Mathematica Hungarica 2008, 120(3):281-299. 10.1007/s10474-008-7139-1 -Bernoulli and Eulerian numbers. Transactions of the American Mathematical Society 1954, 76: 332-350.
-Bernoulli and Eulerian numbers. Transactions of the American Mathematical Society 1954, 76: 332-350. -adic generalized twisted
-adic generalized twisted  -Euler-
-Euler- -function and its applications. Advanced Studies in Contemporary Mathematics 2007, 15(1):37-47.
-function and its applications. Advanced Studies in Contemporary Mathematics 2007, 15(1):37-47. -analogue of the Lerch zeta function. Advanced Studies in Contemporary Mathematics 2006, 12(2):213-223.
-analogue of the Lerch zeta function. Advanced Studies in Contemporary Mathematics 2006, 12(2):213-223. -adic interpolation functions and Kummer-type congruences for
-adic interpolation functions and Kummer-type congruences for  -twisted and
-twisted and  -generalized twisted Euler numbers. Advanced Studies in Contemporary Mathematics 2004, 9(2):203-216.
-generalized twisted Euler numbers. Advanced Studies in Contemporary Mathematics 2004, 9(2):203-216. -adic
-adic  -
- -functions and sums of powers. Discrete Mathematics 2002, 252(1–3):179-187.
-functions and sums of powers. Discrete Mathematics 2002, 252(1–3):179-187. -Volkenborn integration. Russian Journal of Mathematical Physics 2002, 9(3):288-299.
-Volkenborn integration. Russian Journal of Mathematical Physics 2002, 9(3):288-299. -zeta functions and their values at negative integers. Russian Journal of Mathematical Physics 2004, 11(1):71-76.
-zeta functions and their values at negative integers. Russian Journal of Mathematical Physics 2004, 11(1):71-76. -Riemann zeta function. International Journal of Mathematics and Mathematical Sciences 2004, 2004(12):599-605. 10.1155/S0161171204307180
-Riemann zeta function. International Journal of Mathematics and Mathematical Sciences 2004, 2004(12):599-605. 10.1155/S0161171204307180 -analog of the two-variable
-analog of the two-variable  -adic
-adic  -function. Russian Journal of Mathematical Physics 2005, 12(2):186-196.
-function. Russian Journal of Mathematical Physics 2005, 12(2):186-196. -adic
-adic  -
- -functions. Advanced Studies in Contemporary Mathematics 2006, 12(1):61-72.
-functions. Advanced Studies in Contemporary Mathematics 2006, 12(1):61-72. -adic
-adic  -function. Russian Journal of Mathematical Physics 2006, 13(2):151-157. 10.1134/S1061920806020038
-function. Russian Journal of Mathematical Physics 2006, 13(2):151-157. 10.1134/S1061920806020038 -extension of the Euler formula and trigonometric functions. Russian Journal of Mathematical Physics 2007, 14(3):275-278. 10.1134/S1061920807030041
-extension of the Euler formula and trigonometric functions. Russian Journal of Mathematical Physics 2007, 14(3):275-278. 10.1134/S1061920807030041 -adic interpolating function for
-adic interpolating function for  -Euler numbers and its derivatives. Journal of Mathematical Analysis and Applications 2008, 339(1):598-608. 10.1016/j.jmaa.2007.07.027
-Euler numbers and its derivatives. Journal of Mathematical Analysis and Applications 2008, 339(1):598-608. 10.1016/j.jmaa.2007.07.027 -Bernoulli numbers and polynomials associated with Gaussian binomial coefficients. Russian Journal of Mathematical Physics 2008, 15(1):51-57.
-Bernoulli numbers and polynomials associated with Gaussian binomial coefficients. Russian Journal of Mathematical Physics 2008, 15(1):51-57. -Euler numbers and polynomials. Advanced Studies in Contemporary Mathematics 2008, 16(2):161-170.
-Euler numbers and polynomials. Advanced Studies in Contemporary Mathematics 2008, 16(2):161-170. -Bernoulli polynomials. Abstract and Applied Analysis 2008, 2008:-7.
-Bernoulli polynomials. Abstract and Applied Analysis 2008, 2008:-7. -adic invariant integral on
-adic invariant integral on  for Bernoulli and Euler polynomials. Journal of Difference Equations and Applications 2008, 14(12):1267-1277. 10.1080/10236190801943220
for Bernoulli and Euler polynomials. Journal of Difference Equations and Applications 2008, 14(12):1267-1277. 10.1080/10236190801943220 -Euler numbers and polynomials. Proceedings of the Jangjeon Mathematical Society 2006, 9(2):227-232.
-Euler numbers and polynomials. Proceedings of the Jangjeon Mathematical Society 2006, 9(2):227-232. -Volkenborn integration. Proceedings of the Jangjeon Mathematical Society 2005, 8(1):13-17.
-Volkenborn integration. Proceedings of the Jangjeon Mathematical Society 2005, 8(1):13-17. -Riemann zeta function. Proceedings of the Jangjeon Mathematical Society 2004, 7(2):125-127.
-Riemann zeta function. Proceedings of the Jangjeon Mathematical Society 2004, 7(2):125-127. -integers. Advanced Studies in Contemporary Mathematics 2006, 13(2):159-164.
-integers. Advanced Studies in Contemporary Mathematics 2006, 13(2):159-164. -
- -functions associated with Daehee numbers. Russian Journal of Mathematical Physics 2008, 15(1):58-65.
-functions associated with Daehee numbers. Russian Journal of Mathematical Physics 2008, 15(1):58-65. -extension of Apostol-Euler numbers and polynomials. Abstract and Applied Analysis 2008, 2008:-10.
-extension of Apostol-Euler numbers and polynomials. Abstract and Applied Analysis 2008, 2008:-10. -Euler numbers and polynomials associated with
-Euler numbers and polynomials associated with  -adic
-adic  -integrals. Advances in Difference Equations 2008, 2008:-11.
-integrals. Advances in Difference Equations 2008, 2008:-11. -Euler numbers and their applications. Abstract and Applied Analysis 2008, 2008:-16.
-Euler numbers and their applications. Abstract and Applied Analysis 2008, 2008:-16. -Euler numbers and polynomials related to their interpolation functions. Applied Mathematics Letters 2008, 21(9):934-939. 10.1016/j.aml.2007.10.005
-Euler numbers and polynomials related to their interpolation functions. Applied Mathematics Letters 2008, 21(9):934-939. 10.1016/j.aml.2007.10.005 -adic
-adic  -Euler measure. General Mathematics 2007, 15(2):24-37.
-Euler measure. General Mathematics 2007, 15(2):24-37. -adic
-adic  -Euler measure. Advanced Studies in Contemporary Mathematics 2007, 14(2):233-239.
-Euler measure. Advanced Studies in Contemporary Mathematics 2007, 14(2):233-239. -analogue of Euler-Barnes' polynomials. Advanced Studies in Contemporary Mathematics 2004, 9(2):153-163.
-analogue of Euler-Barnes' polynomials. Advanced Studies in Contemporary Mathematics 2004, 9(2):153-163. -function and twisted Bernoulli numbers. Advanced Studies in Contemporary Mathematics 2005, 11(2):205-218.
-function and twisted Bernoulli numbers. Advanced Studies in Contemporary Mathematics 2005, 11(2):205-218. -adic twisted
-adic twisted  -
- -functions related to generalized twisted Bernoulli numbers. Russian Journal of Mathematical Physics 2006, 13(3):340-348. 10.1134/S1061920806030095
-functions related to generalized twisted Bernoulli numbers. Russian Journal of Mathematical Physics 2006, 13(3):340-348. 10.1134/S1061920806030095 -Bernoulli numbers and polynomials associated with multiple
-Bernoulli numbers and polynomials associated with multiple  -zeta functions and basic
-zeta functions and basic  -series. Russian Journal of Mathematical Physics 2005, 12(2):241-268.
-series. Russian Journal of Mathematical Physics 2005, 12(2):241-268.