- Research Article
- Open access
- Published:
Multiple Twisted 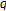 -Euler Numbers and Polynomials Associated with
-Euler Numbers and Polynomials Associated with  -Adic
-Adic  -Integrals
-Integrals
Advances in Difference Equations volume 2008, Article number: 738603 (2008)
Abstract
By using  -adic
-adic  -integrals on
-integrals on  , we define multiple twisted
, we define multiple twisted  -Euler numbers and polynomials. We also find Witt's type formula for multiple twisted
-Euler numbers and polynomials. We also find Witt's type formula for multiple twisted  -Euler numbers and discuss some characterizations of multiple twisted
-Euler numbers and discuss some characterizations of multiple twisted  -Euler Zeta functions. In particular, we construct multiple twisted Barnes' type
-Euler Zeta functions. In particular, we construct multiple twisted Barnes' type  -Euler polynomials and multiple twisted Barnes' type
-Euler polynomials and multiple twisted Barnes' type  -Euler Zeta functions. Finally, we define multiple twisted Dirichlet's type
-Euler Zeta functions. Finally, we define multiple twisted Dirichlet's type  -Euler numbers and polynomials, and give Witt's type formula for them.
-Euler numbers and polynomials, and give Witt's type formula for them.
1. Introduction
Let  be a fixed odd prime number. Throughout this paper,
be a fixed odd prime number. Throughout this paper,  ,
,  , and
, and  are, respectively, the ring of
are, respectively, the ring of  -adic rational integers, the field of
-adic rational integers, the field of  -adic rational numbers, and the
-adic rational numbers, and the  -adic completion of the algebraic closure of
-adic completion of the algebraic closure of  . The
. The  -adic absolute value in
-adic absolute value in  is normalized so that
is normalized so that  . When one talks about
. When one talks about  -extension,
-extension,  is variously considered as an indeterminate, a complex number,
is variously considered as an indeterminate, a complex number,  or a
or a  -adic number
-adic number  . If
. If  , one normally assumes that
, one normally assumes that  . If
. If  , one normally assumes that
, one normally assumes that  so that
so that  for each
for each  . We use the notations
. We use the notations

(cf. [1–14]), for all  . For a fixed odd positive integer
. For a fixed odd positive integer  with
with  , set
, set

where  lies in
lies in  . For any
. For any  ,
,

is known to be a distribution on  (cf. [1–28]).
(cf. [1–28]).
We say that  is uniformly differentiable function at a point
is uniformly differentiable function at a point  and denote this property by
and denote this property by  if the difference quotients
if the difference quotients

have a limit  as
as  (cf. [25]).
(cf. [25]).
The  -adic
-adic  -integral of a function
-integral of a function  was defined as
was defined as


(cf. [4, 24, 25, 28]), from (1.6), we derive

where  . If we take
. If we take  , then we have
, then we have  . From (1.7), we obtain that
. From (1.7), we obtain that

In Section 2, we define the multiple twisted  -Euler numbers and polynomials on
-Euler numbers and polynomials on  and find Witt's type formula for multiple twisted
and find Witt's type formula for multiple twisted  -Euler numbers. We also have sums of consecutive multiple twisted
-Euler numbers. We also have sums of consecutive multiple twisted  -Euler numbers. In Section 3, we consider multiple twisted
-Euler numbers. In Section 3, we consider multiple twisted  -Euler Zeta functions which interpolate new multiple twisted
-Euler Zeta functions which interpolate new multiple twisted  -Euler polynomials at negative integers and investigate some characterizations of them. In Section 4, we construct the multiple twisted Barnes' type
-Euler polynomials at negative integers and investigate some characterizations of them. In Section 4, we construct the multiple twisted Barnes' type  -Euler polynomials and multiple twisted Barnes' type
-Euler polynomials and multiple twisted Barnes' type  -Euler Zeta functions which interpolate new multiple twisted Barnes' type
-Euler Zeta functions which interpolate new multiple twisted Barnes' type  -Euler polynomials at negative integers. In Section 5, we define multiple twisted Dirichlet's type
-Euler polynomials at negative integers. In Section 5, we define multiple twisted Dirichlet's type  -Euler numbers and polynomials and give Witt's type formula for them.
-Euler numbers and polynomials and give Witt's type formula for them.
2. Multiple Twisted 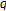 -Euler Numbers and Polynomials
-Euler Numbers and Polynomials
In this section, we assume that  with
with  . For
. For  , by the definition of
, by the definition of  -adic
-adic  -integral on
-integral on  , we have
, we have

where  . If
. If  is odd positive integer, we have
is odd positive integer, we have

Let  be the locally constant space, where
be the locally constant space, where  is the cyclic group of order
is the cyclic group of order  . For
. For  , we denote the locally constant function by
, we denote the locally constant function by

(cf. [5, 7–14, 16, 18]). If we take  , then we have
, then we have

Now we define the twisted  -Euler numbers
-Euler numbers  as follows:
as follows:

We note that by substituting  ,
,  are the familiar Euler numbers. Over five decades ago, Carlitz defined
are the familiar Euler numbers. Over five decades ago, Carlitz defined  -extension of Euler numbers (cf. [15]). From (2.4) and (2.5), we note that Witt's type formula for a twisted
-extension of Euler numbers (cf. [15]). From (2.4) and (2.5), we note that Witt's type formula for a twisted  -Euler number is given by
-Euler number is given by

for each  and
and  .
.
Twisted  -Euler polynomials
-Euler polynomials  are defined by means of the generating function
are defined by means of the generating function

where  . By using the
. By using the  th iterative fermionic
th iterative fermionic  -adic
-adic  -integral on
-integral on  , we define multiple twisted
, we define multiple twisted  -Euler number as follows:
-Euler number as follows:

Thus we give Witt's type formula for multiple twisted  -Euler numbers as follows.
-Euler numbers as follows.
Theorem 2.1.
For each  and
and  ,
,

where

From (2.8) and (2.9), we obtain the following theorem.
Theorem 2.2.
For  and
and  ,
,

From these formulas, we consider multivariate fermionic  -adic
-adic  -integral on
-integral on  as follows:
as follows:

Then we can define the multiple twisted  -Euler polynomials
-Euler polynomials  as follows:
as follows:

From (2.12) and (2.13), we note that

Then by the  th differentiation on both sides of (2.14), we obtain the following.
th differentiation on both sides of (2.14), we obtain the following.
Theorem 2.3.
For each  and
and  ,
,

Note that

Then we see that

From (2.15) and (2.17), we obtain the sums of powers of consecutive  -Euler numbers as follows.
-Euler numbers as follows.
Theorem 2.4.
For each  and
and  ,
,

3. Multiple Twisted 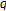 -Euler Zeta Functions
-Euler Zeta Functions
For  with
with  and
and  , the multiple twisted
, the multiple twisted  -Euler numbers can be considered as follows:
-Euler numbers can be considered as follows:

From (3.1), we note that

By the  th differentiation on both sides of (3.2) at
th differentiation on both sides of (3.2) at  , we obtain that
, we obtain that

From (3.3), we derive multiple twisted  -Euler Zeta function as follows:
-Euler Zeta function as follows:

for all  . We also obtain the following theorem in which multiple twisted
. We also obtain the following theorem in which multiple twisted  -Euler Zeta functions interpolate multiple twisted
-Euler Zeta functions interpolate multiple twisted  -Euler polynomials.
-Euler polynomials.
Theorem 3.1.
For  and
and  ,
,

4. Multiple Twisted Barnes' Type 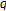 -Euler Polynomials
-Euler Polynomials
In this section, we consider the generating function of multiple twisted  -Euler polynomials:
-Euler polynomials:

We note that

By the  th differentiation on both sides of (4.2) at
th differentiation on both sides of (4.2) at  , we obtain that
, we obtain that

Thus we can consider multiple twisted Hurwitz's type  -Euler Zeta function as follows:
-Euler Zeta function as follows:

for all  and
and  . We note that
. We note that  is analytic function in the whole complex
is analytic function in the whole complex  -plane and
-plane and  . We also remark that if
. We also remark that if  and
and  , then
, then  is Hurwitz's type
is Hurwitz's type  -Euler Zeta function (see [7, 27]). The following theorem means that multiple twisted
-Euler Zeta function (see [7, 27]). The following theorem means that multiple twisted  -Euler Zeta functions interpolate multiple twisted
-Euler Zeta functions interpolate multiple twisted  -Euler polynomials at negative integers.
-Euler polynomials at negative integers.
Theorem 4.1.
For  ,
,  ,
,  , and
, and  ,
,

Let us consider

where  and
and  . Then
. Then  will be called multiple twisted Barnes' type
will be called multiple twisted Barnes' type  -Euler polynomials. We note that
-Euler polynomials. We note that

By the  th differentiation of both sides of (4.6), we obtain the following theorem.
th differentiation of both sides of (4.6), we obtain the following theorem.
Theorem 4.2.
For each  ,
,  ,
,  , and
, and  ,
,

where

From (4.8), we consider multiple twisted Barnes' type  -Euler Zeta function defined as follows: for each
-Euler Zeta function defined as follows: for each  ,
,  ,
,  , and
, and  ,
,

We note that  is analytic function in the whole complex
is analytic function in the whole complex  -plane. We also see that multiple twisted Barnes' type
-plane. We also see that multiple twisted Barnes' type  -Euler Zeta functions interpolate multiple twisted Barnes' type
-Euler Zeta functions interpolate multiple twisted Barnes' type  -Euler polynomials at negative integers as follows.
-Euler polynomials at negative integers as follows.
Theorem 4.3.
For each  ,
,  ,
,  , and
, and  ,
,

5. Multiple Twisted Dirichlet's Type 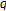 -Euler Numbers and Polynomials
-Euler Numbers and Polynomials
Let  be a Dirichlet's character with conductor
be a Dirichlet's character with conductor  and
and  . If we take
. If we take  , then we have
, then we have  . From (2.2), we derive
. From (2.2), we derive

In view of (5.1), we can define twisted Dirichlet's type  -Euler numbers as follows:
-Euler numbers as follows:

(cf. [17, 19, 21, 22]). From (5.1) and (5.2), we can give Witt's type formula for twisted Dirichlet's type  -Euler numbers as follows.
-Euler numbers as follows.
Theorem 5.1.
Let  be a Dirichlet's character with conductor
be a Dirichlet's character with conductor  . For each
. For each  ,
,  , we have
, we have

We note that if  , then
, then  is the generalized
is the generalized  -Euler numbers attached to
-Euler numbers attached to  (see [18, 26]). From (5.2), we also see that
(see [18, 26]). From (5.2), we also see that

By (5.2) and (5.4), we obtain that

From (5.5), we can define the  -function as follows:
-function as follows:

for all  . We note that
. We note that  is analytic function in the whole complex
is analytic function in the whole complex  -plane. From (5.5) and (5.6), we can derive the following result.
-plane. From (5.5) and (5.6), we can derive the following result.
Theorem 5.2.
Let  be a Dirichlet's character with conductor
be a Dirichlet's character with conductor  . For each
. For each  ,
,  , we have
, we have

Now, in view of (5.1), we can define multiple twisted Dirichlet's type  -Euler numbers by means of the generating function as follows:
-Euler numbers by means of the generating function as follows:

where  . We note that if
. We note that if  , then
, then  is a multiple generalized
is a multiple generalized  -Euler number (see [22]).
-Euler number (see [22]).
By using the same method used in (2.8) and (2.9),

From (5.9), we can give Witt's type formula for multiple twisted Dirichlet's type  -Euler numbers.
-Euler numbers.
Theorem 5.3.
Let  be a Dirichlet's character with conductor
be a Dirichlet's character with conductor  . For each
. For each  ,
,  , and
, and  , we have
, we have

where  and
and

From (5.10), we also obtain the sums of powers of consecutive multiple twisted Dirichlet's type  -Euler numbers as follows.
-Euler numbers as follows.
Theorem 5.4.
Let  be a Dirichlet's character with conductor
be a Dirichlet's character with conductor  . For each
. For each  ,
,  , and
, and  , we have
, we have

Finally, we consider multiple twisted Dirichlet's type  -Euler polynomials defined by means of the generating functions as follows:
-Euler polynomials defined by means of the generating functions as follows:

where  and
and  . From (5.13), we note that
. From (5.13), we note that

Clearly, we obtain the following two theorems.
Theorem 5.5.
Let  be a Dirichlet's character with conductor
be a Dirichlet's character with conductor  . For each
. For each  ,
,  ,
,  , and
, and  , we have
, we have

where

Theorem 5.6.
Let  be a Dirichlet's character with conductor
be a Dirichlet's character with conductor  . For each
. For each  ,
,  ,
,  , and
, and  , we have
, we have

References
Kim T:Analytic continuation of multiple
 -zeta functions and their values at negative integers. Russian Journal of Mathematical Physics 2004, 11(1):71-76.
-zeta functions and their values at negative integers. Russian Journal of Mathematical Physics 2004, 11(1):71-76.Kim T:On the analogs of Euler numbers and polynomials associated with
 -adic
-adic  -integral on
-integral on 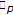 at
at 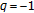 . Journal of Mathematical Analysis and Applications 2007, 331(2):779-792. 10.1016/j.jmaa.2006.09.027
. Journal of Mathematical Analysis and Applications 2007, 331(2):779-792. 10.1016/j.jmaa.2006.09.027Kim T:A note on some formulae for the
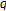 -Euler numbers and polynomials. Proceedings of the Jangjeon Mathematical Society 2006, 9(2):227-232.
-Euler numbers and polynomials. Proceedings of the Jangjeon Mathematical Society 2006, 9(2):227-232.Kim T, Kim M-S, Jang L-C, Rim S-H:New
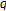 -Euler numbers and polynomials associated with
-Euler numbers and polynomials associated with  -adic
-adic 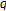 -integrals. Advanced Studies in Contemporary Mathematics 2007, 15(2):243-252.
-integrals. Advanced Studies in Contemporary Mathematics 2007, 15(2):243-252.Jang L-C:On a
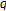 -analogue of the
-analogue of the  -adic generalized twisted
-adic generalized twisted  -functions and
-functions and  -adic
-adic  -integrals. Journal of the Korean Mathematical Society 2007, 44(1):1-10.
-integrals. Journal of the Korean Mathematical Society 2007, 44(1):1-10.Jang L-C, Kim S-D, Park D-W, Ro Y-S: A note on Euler number and polynomials. Journal of Inequalities and Applications 2006, 2006:-5.
Ozden H, Simsek Y:A new extension of
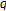 -Euler numbers and polynomials related to their interpolation functions. Applied mathematics Letters. In press
-Euler numbers and polynomials related to their interpolation functions. Applied mathematics Letters. In pressOzden H, Simsek Y, Cangul IN:Remarks on sum of products of
 v-twisted Euler polynomials and numbers. Journal of Inequalities and Applications 2008, 2008:-8.
v-twisted Euler polynomials and numbers. Journal of Inequalities and Applications 2008, 2008:-8.Ozden H, Simsek Y, Cangul IN:Multivariate interpolation functions of higher order
 -Euler numbers and their applications. Abstract and Applied Analysis. In press
-Euler numbers and their applications. Abstract and Applied Analysis. In pressSimsek Y:Twisted
 -Bernoulli numbers and polynomials related to twisted
-Bernoulli numbers and polynomials related to twisted 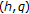 -zeta function and
-zeta function and 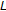 -function. Journal of Mathematical Analysis and Applications 2006, 324(2):790-804. 10.1016/j.jmaa.2005.12.057
-function. Journal of Mathematical Analysis and Applications 2006, 324(2):790-804. 10.1016/j.jmaa.2005.12.057Simsek Y:On
 -adic twisted
-adic twisted 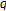 -
-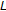 -functions related to generalized twisted Bernoulli numbers. Russian Journal of Mathematical Physics 2006, 13(3):340-348. 10.1134/S1061920806030095
-functions related to generalized twisted Bernoulli numbers. Russian Journal of Mathematical Physics 2006, 13(3):340-348. 10.1134/S1061920806030095Simsek Y:On twisted
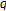 -Hurwitz zeta function and
-Hurwitz zeta function and  -two-variable
-two-variable 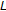 -function. Applied Mathematics and Computation 2007, 187(1):466-473. 10.1016/j.amc.2006.08.146
-function. Applied Mathematics and Computation 2007, 187(1):466-473. 10.1016/j.amc.2006.08.146Simsek Y:The behavior of the twisted
 -adic
-adic 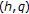 -
- -functions at
-functions at 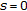 . Journal of the Korean Mathematical Society 2007, 44(4):915-929. 10.4134/JKMS.2007.44.4.915
. Journal of the Korean Mathematical Society 2007, 44(4):915-929. 10.4134/JKMS.2007.44.4.915Simsek Y, Kurt V, Kim D:New approach to the complete sum of products of the twisted
 -Bernoulli numbers and polynomials. Journal of Nonlinear Mathematical Physics 2007, 14(1):44-56. 10.2991/jnmp.2007.14.1.5
-Bernoulli numbers and polynomials. Journal of Nonlinear Mathematical Physics 2007, 14(1):44-56. 10.2991/jnmp.2007.14.1.5Carlitz L:
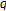 -Bernoulli numbers and polynomials. Duke Mathematical Journal 1948, 15(4):987-1000. 10.1215/S0012-7094-48-01588-9
-Bernoulli numbers and polynomials. Duke Mathematical Journal 1948, 15(4):987-1000. 10.1215/S0012-7094-48-01588-9Cenkci M:The
 -adic generalized twisted
-adic generalized twisted  -Euler-
-Euler-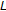 -function and its applications. Advanced Studies in Contemporary Mathematics 2007, 15(1):37-47.
-function and its applications. Advanced Studies in Contemporary Mathematics 2007, 15(1):37-47.Cenkci M, Can M:Some results on
 -analogue of the Lerch zeta function. Advanced Studies in Contemporary Mathematics 2006, 12(2):213-223.
-analogue of the Lerch zeta function. Advanced Studies in Contemporary Mathematics 2006, 12(2):213-223.Cenkci M, Can M, Kurt V:
 -adic interpolation functions and Kummer-type congruences for
-adic interpolation functions and Kummer-type congruences for 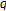 -twisted and
-twisted and 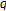 -generalized twisted Euler numbers. Advanced Studies in Contemporary Mathematics 2004, 9(2):203-216.
-generalized twisted Euler numbers. Advanced Studies in Contemporary Mathematics 2004, 9(2):203-216.Cenkci M, Simsek Y, Kurt V:Further remarks on multiple
 -adic
-adic  -
- -function of two variables. Advanced Studies in Contemporary Mathematics 2007, 14(1):49-68.
-function of two variables. Advanced Studies in Contemporary Mathematics 2007, 14(1):49-68.Kim T: On Euler-Barnes multiple zeta functions. Russian Journal of Mathematical Physics 2003, 10(3):261-267.
Kim T:Power series and asymptotic series associated with the
 -analog of the two-variable
-analog of the two-variable  -adic
-adic  -function. Russian Journal of Mathematical Physics 2005, 12(2):186-196.
-function. Russian Journal of Mathematical Physics 2005, 12(2):186-196.Kim T:Multiple
 -adic
-adic  -function. Russian Journal of Mathematical Physics 2006, 13(2):151-157. 10.1134/S1061920806020038
-function. Russian Journal of Mathematical Physics 2006, 13(2):151-157. 10.1134/S1061920806020038Kim T:A note on
 -adic
-adic  -integral on
-integral on  associated with
associated with 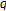 -Euler numbers. Advanced Studies in Contemporary Mathematics 2007, 15(2):133-137.
-Euler numbers. Advanced Studies in Contemporary Mathematics 2007, 15(2):133-137.Kim T:
 -Euler numbers and polynomials associated with
-Euler numbers and polynomials associated with  -adic
-adic  -integrals. Journal of Nonlinear Mathematical Physics 2007, 14(1):15-27. 10.2991/jnmp.2007.14.1.3
-integrals. Journal of Nonlinear Mathematical Physics 2007, 14(1):15-27. 10.2991/jnmp.2007.14.1.3Kim T:An invariant
 -adic
-adic  -integral on
-integral on  . Applied Mathematics Letters 2008, 21(2):105-108. 10.1016/j.aml.2006.11.011
. Applied Mathematics Letters 2008, 21(2):105-108. 10.1016/j.aml.2006.11.011Kim T:On
 -adic interpolating function for
-adic interpolating function for 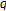 -Euler numbers and its derivatives. Journal of Mathematical Analysis and Applications 2008, 339(1):598-608. 10.1016/j.jmaa.2007.07.027
-Euler numbers and its derivatives. Journal of Mathematical Analysis and Applications 2008, 339(1):598-608. 10.1016/j.jmaa.2007.07.027Kim T, Rim S-H:New Changhee
 -Euler numbers and polynomials associated with
-Euler numbers and polynomials associated with  -adic
-adic 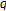 -integrals. Computers & Mathematics with Applications 2007, 54(4):484-489. 10.1016/j.camwa.2006.12.028
-integrals. Computers & Mathematics with Applications 2007, 54(4):484-489. 10.1016/j.camwa.2006.12.028Kim T, Choi JY, Sug JY:Extended
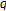 -Euler numbers and polynomials associated with fermionic
-Euler numbers and polynomials associated with fermionic  -adic
-adic 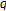 -integral on
-integral on 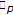 . Russian Journal of Mathematical Physics 2007, 14(2):160-163. 10.1134/S1061920807020045
. Russian Journal of Mathematical Physics 2007, 14(2):160-163. 10.1134/S1061920807020045
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Jang, LC. Multiple Twisted 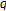 -Euler Numbers and Polynomials Associated with
-Euler Numbers and Polynomials Associated with  -Adic
-Adic  -Integrals.
Adv Differ Equ 2008, 738603 (2008). https://doi.org/10.1155/2008/738603
-Integrals.
Adv Differ Equ 2008, 738603 (2008). https://doi.org/10.1155/2008/738603
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1155/2008/738603
 -Euler Numbers and Polynomials
-Euler Numbers and Polynomials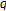 -Euler Zeta Functions
-Euler Zeta Functions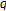 -Euler Polynomials
-Euler Polynomials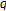 -Euler Numbers and Polynomials
-Euler Numbers and Polynomials -zeta functions and their values at negative integers. Russian Journal of Mathematical Physics 2004, 11(1):71-76.
-zeta functions and their values at negative integers. Russian Journal of Mathematical Physics 2004, 11(1):71-76. -adic
-adic  -integral on
-integral on 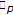 at
at 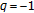 . Journal of Mathematical Analysis and Applications 2007, 331(2):779-792. 10.1016/j.jmaa.2006.09.027
. Journal of Mathematical Analysis and Applications 2007, 331(2):779-792. 10.1016/j.jmaa.2006.09.027 -Euler numbers and polynomials. Proceedings of the Jangjeon Mathematical Society 2006, 9(2):227-232.
-Euler numbers and polynomials. Proceedings of the Jangjeon Mathematical Society 2006, 9(2):227-232. -Euler numbers and polynomials associated with
-Euler numbers and polynomials associated with  -adic
-adic  -integrals. Advanced Studies in Contemporary Mathematics 2007, 15(2):243-252.
-integrals. Advanced Studies in Contemporary Mathematics 2007, 15(2):243-252.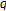 -analogue of the
-analogue of the  -adic generalized twisted
-adic generalized twisted  -functions and
-functions and  -adic
-adic  -integrals. Journal of the Korean Mathematical Society 2007, 44(1):1-10.
-integrals. Journal of the Korean Mathematical Society 2007, 44(1):1-10.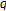 -Euler numbers and polynomials related to their interpolation functions. Applied mathematics Letters. In press
-Euler numbers and polynomials related to their interpolation functions. Applied mathematics Letters. In press v-twisted Euler polynomials and numbers. Journal of Inequalities and Applications 2008, 2008:-8.
v-twisted Euler polynomials and numbers. Journal of Inequalities and Applications 2008, 2008:-8. -Euler numbers and their applications. Abstract and Applied Analysis. In press
-Euler numbers and their applications. Abstract and Applied Analysis. In press -Bernoulli numbers and polynomials related to twisted
-Bernoulli numbers and polynomials related to twisted 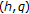 -zeta function and
-zeta function and 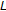 -function. Journal of Mathematical Analysis and Applications 2006, 324(2):790-804. 10.1016/j.jmaa.2005.12.057
-function. Journal of Mathematical Analysis and Applications 2006, 324(2):790-804. 10.1016/j.jmaa.2005.12.057 -adic twisted
-adic twisted  -
- -functions related to generalized twisted Bernoulli numbers. Russian Journal of Mathematical Physics 2006, 13(3):340-348. 10.1134/S1061920806030095
-functions related to generalized twisted Bernoulli numbers. Russian Journal of Mathematical Physics 2006, 13(3):340-348. 10.1134/S1061920806030095 -Hurwitz zeta function and
-Hurwitz zeta function and  -two-variable
-two-variable  -function. Applied Mathematics and Computation 2007, 187(1):466-473. 10.1016/j.amc.2006.08.146
-function. Applied Mathematics and Computation 2007, 187(1):466-473. 10.1016/j.amc.2006.08.146 -adic
-adic 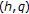 -
- -functions at
-functions at 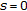 . Journal of the Korean Mathematical Society 2007, 44(4):915-929. 10.4134/JKMS.2007.44.4.915
. Journal of the Korean Mathematical Society 2007, 44(4):915-929. 10.4134/JKMS.2007.44.4.915 -Bernoulli numbers and polynomials. Journal of Nonlinear Mathematical Physics 2007, 14(1):44-56. 10.2991/jnmp.2007.14.1.5
-Bernoulli numbers and polynomials. Journal of Nonlinear Mathematical Physics 2007, 14(1):44-56. 10.2991/jnmp.2007.14.1.5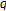 -Bernoulli numbers and polynomials. Duke Mathematical Journal 1948, 15(4):987-1000. 10.1215/S0012-7094-48-01588-9
-Bernoulli numbers and polynomials. Duke Mathematical Journal 1948, 15(4):987-1000. 10.1215/S0012-7094-48-01588-9 -adic generalized twisted
-adic generalized twisted  -Euler-
-Euler-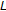 -function and its applications. Advanced Studies in Contemporary Mathematics 2007, 15(1):37-47.
-function and its applications. Advanced Studies in Contemporary Mathematics 2007, 15(1):37-47. -analogue of the Lerch zeta function. Advanced Studies in Contemporary Mathematics 2006, 12(2):213-223.
-analogue of the Lerch zeta function. Advanced Studies in Contemporary Mathematics 2006, 12(2):213-223. -adic interpolation functions and Kummer-type congruences for
-adic interpolation functions and Kummer-type congruences for 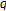 -twisted and
-twisted and 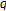 -generalized twisted Euler numbers. Advanced Studies in Contemporary Mathematics 2004, 9(2):203-216.
-generalized twisted Euler numbers. Advanced Studies in Contemporary Mathematics 2004, 9(2):203-216. -adic
-adic  -
-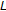 -function of two variables. Advanced Studies in Contemporary Mathematics 2007, 14(1):49-68.
-function of two variables. Advanced Studies in Contemporary Mathematics 2007, 14(1):49-68.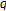 -analog of the two-variable
-analog of the two-variable  -adic
-adic  -function. Russian Journal of Mathematical Physics 2005, 12(2):186-196.
-function. Russian Journal of Mathematical Physics 2005, 12(2):186-196. -adic
-adic  -function. Russian Journal of Mathematical Physics 2006, 13(2):151-157. 10.1134/S1061920806020038
-function. Russian Journal of Mathematical Physics 2006, 13(2):151-157. 10.1134/S1061920806020038 -adic
-adic  -integral on
-integral on  associated with
associated with  -Euler numbers. Advanced Studies in Contemporary Mathematics 2007, 15(2):133-137.
-Euler numbers. Advanced Studies in Contemporary Mathematics 2007, 15(2):133-137. -Euler numbers and polynomials associated with
-Euler numbers and polynomials associated with  -adic
-adic  -integrals. Journal of Nonlinear Mathematical Physics 2007, 14(1):15-27. 10.2991/jnmp.2007.14.1.3
-integrals. Journal of Nonlinear Mathematical Physics 2007, 14(1):15-27. 10.2991/jnmp.2007.14.1.3 -adic
-adic  -integral on
-integral on  . Applied Mathematics Letters 2008, 21(2):105-108. 10.1016/j.aml.2006.11.011
. Applied Mathematics Letters 2008, 21(2):105-108. 10.1016/j.aml.2006.11.011 -adic interpolating function for
-adic interpolating function for  -Euler numbers and its derivatives. Journal of Mathematical Analysis and Applications 2008, 339(1):598-608. 10.1016/j.jmaa.2007.07.027
-Euler numbers and its derivatives. Journal of Mathematical Analysis and Applications 2008, 339(1):598-608. 10.1016/j.jmaa.2007.07.027 -Euler numbers and polynomials associated with
-Euler numbers and polynomials associated with  -adic
-adic 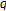 -integrals. Computers & Mathematics with Applications 2007, 54(4):484-489. 10.1016/j.camwa.2006.12.028
-integrals. Computers & Mathematics with Applications 2007, 54(4):484-489. 10.1016/j.camwa.2006.12.028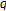 -Euler numbers and polynomials associated with fermionic
-Euler numbers and polynomials associated with fermionic  -adic
-adic 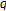 -integral on
-integral on 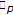 . Russian Journal of Mathematical Physics 2007, 14(2):160-163. 10.1134/S1061920807020045
. Russian Journal of Mathematical Physics 2007, 14(2):160-163. 10.1134/S1061920807020045