- Research Article
- Open access
- Published:
Value Distributions and Uniqueness of Difference Polynomials
Advances in Difference Equations volume 2011, Article number: 234215 (2011)
Abstract
We investigate the zeros distributions of difference polynomials of meromorphic functions, which can be viewed as the Hayman conjecture as introduced by (Hayman 1967) for difference. And we also study the uniqueness of difference polynomials of meromorphic functions sharing a common value, and obtain uniqueness theorems for difference.
1. Introduction
A meromorphic function means meromorphic in the whole complex plane. Given a meromorphic function  , recall that
, recall that  ,
,  is a small function with respect to
is a small function with respect to  , if
, if  , where
, where  is used to denote any quantity satisfying
is used to denote any quantity satisfying  , as
, as  outside a possible exceptional set of finite logarithmic measure. We use notations
outside a possible exceptional set of finite logarithmic measure. We use notations  ,
,  to denote the order of growth of
to denote the order of growth of  and the exponent of convergence of the poles of
and the exponent of convergence of the poles of  , respectively. We say that meromorphic functions
, respectively. We say that meromorphic functions  and
and  share a finite value
share a finite value  IM (ignoring multiplicities) when
IM (ignoring multiplicities) when  and
and  have the same zeros. If
have the same zeros. If  and
and  have the same zeros with the same multiplicities, then we say that
have the same zeros with the same multiplicities, then we say that  and
and  share the value
share the value  CM (counting multiplicities). We assume that the reader is familiar with standard notations and fundamental results of Nevanlinna Theory [1–3].
CM (counting multiplicities). We assume that the reader is familiar with standard notations and fundamental results of Nevanlinna Theory [1–3].
As we all know that a finite value  is called the Picard exception value of
is called the Picard exception value of  , if
, if  has no zeros. The Picard theorem shows that a transcendental entire function has at most one Picard exception value, a transcendental meromorphic function has at most two Picard exception values. The Hayman conjecture [4], is that if
has no zeros. The Picard theorem shows that a transcendental entire function has at most one Picard exception value, a transcendental meromorphic function has at most two Picard exception values. The Hayman conjecture [4], is that if  is a transcendental meromorphic function and
is a transcendental meromorphic function and  , then
, then  takes every finite nonzero value infinitely often. This conjecture has been solved by Hayman [5] for
takes every finite nonzero value infinitely often. This conjecture has been solved by Hayman [5] for  , by Mues [6] for
, by Mues [6] for  , by Bergweiler and Eremenko [7] for
, by Bergweiler and Eremenko [7] for  . From above, it is showed that the Picard exception value of
. From above, it is showed that the Picard exception value of  may only be zero. Recently, for an analog of Hayman conjecture for difference, Laine and Yang [8, Theorem 2] proved the following.
may only be zero. Recently, for an analog of Hayman conjecture for difference, Laine and Yang [8, Theorem 2] proved the following.
Theorem A.
Let  be a transcendental entire function with finite order and
be a transcendental entire function with finite order and  be a nonzero complex constant. Then for
be a nonzero complex constant. Then for  ,
,  assumes every nonzero value
assumes every nonzero value  infinitely often.
infinitely often.
Remark 1.1.
Theorem A implies that the Picard exception value of  cannot be nonzero constant. However, Theorem A does not remain valid for meromorphic functions. For example,
cannot be nonzero constant. However, Theorem A does not remain valid for meromorphic functions. For example,  ,
,  ,
,  . Thus, we get that
. Thus, we get that  never takes the value −1, and
never takes the value −1, and  never takes the value 1.
never takes the value 1.
As the improvement of Theorem A to the case of meromorphic functions, we first obtain the following theorem. In the following, we assume that  and
and  are small functions with respect of
are small functions with respect of  , unless otherwise specified.
, unless otherwise specified.
Theorem 1.2.
Let  be a transcendental meromorphic function with finite order and
be a transcendental meromorphic function with finite order and  be a nonzero complex constant. If
be a nonzero complex constant. If  , then the difference polynomial
, then the difference polynomial  has infinitely many zeros.
has infinitely many zeros.
Remark 1.3.
The restriction of finite order in Theorem 1.2 cannot be deleted. This can be seen by taking  ,
,  ,
,  is a nonconstant polynomial, and
is a nonconstant polynomial, and  is a nonzero rational function. Then
is a nonzero rational function. Then  is of infinite order and has finitely many poles, while
is of infinite order and has finitely many poles, while

has finitely many zeros. We have given the example when  in Remark 1.1 to show that
in Remark 1.1 to show that  may have finitely many zeros. But we have not succeed in reducing the condition
may have finitely many zeros. But we have not succeed in reducing the condition  to
to  in Theorem 1.2.
in Theorem 1.2.
In the following, we will consider the zeros of other difference polynomials. Using the similar method of the proof of Theorem 1.2 below, we also can obtain the following results.
Theorem 1.4.
Let  be a transcendental meromorphic function with finite order and
be a transcendental meromorphic function with finite order and  be a nonzero complex constant. If
be a nonzero complex constant. If  , then the difference polynomial
, then the difference polynomial  has infinitely many zeros.
has infinitely many zeros.
Theorem 1.5.
Let  be a transcendental meromorphic function with finite order and
be a transcendental meromorphic function with finite order and  be a nonzero complex constant. If
be a nonzero complex constant. If  ,
,  , then the difference polynomial
, then the difference polynomial  has infinitely many zeros.
has infinitely many zeros.
Remark 1.6.
The above two theorems also are not true when  is of infinite order, which can be seen by function
is of infinite order, which can be seen by function  ,
,  , where
, where  in Theorem 1.4 and
in Theorem 1.4 and  in Theorem 1.5.
in Theorem 1.5.
Theorem 1.7.
Let  be a transcendental meromorphic function with finite order and
be a transcendental meromorphic function with finite order and  be a nonzero complex constant. If
be a nonzero complex constant. If  ,
,  , then the difference polynomial
, then the difference polynomial  has infinitely many zeros.
has infinitely many zeros.
Corollary 1.8.
There is no transcendental finite order meromorphic solution of the nonlinear difference equation

where  and
and  ,
,  are rational functions.
are rational functions.
Remark 1.9.
Some results about the zeros distributions of difference polynomials of entire functions or meromorphic functions with the condition  can be found in [9–12]. Theorem 1.7 is a partial improvement of [11, Theorem 1.1] for
can be found in [9–12]. Theorem 1.7 is a partial improvement of [11, Theorem 1.1] for  is an entire function and is also an improvement of [13, Theorem 1.1] for the case of
is an entire function and is also an improvement of [13, Theorem 1.1] for the case of  .
.
The uniqueness problem of differential polynomials of meromorphic functions has been considered by many authors, such as Fang and Hua [14], Qiu and Fang [15], Xu and Yi [16], Yang and Hua [17], and Lahiri and Rupa [18]. The uniqueness results for difference polynomials of entire functions was considered in a recent paper [15], which can be stated as follows.
Theorem B (see [19, Theorem 1.1]).
Let  and
and  be transcendental entire functions with finite order, and
be transcendental entire functions with finite order, and  be a nonzero complex constant. If
be a nonzero complex constant. If  ,
,  and
and  share
share  CM, then
CM, then  for a constant
for a constant  that satisfies
that satisfies  .
.
Theorem C (see [19, Theorem 1.2]).
Let  and
and  be transcendental entire functions with finite order, and
be transcendental entire functions with finite order, and  be a nonzero complex constant. If
be a nonzero complex constant. If  ,
,  and
and  share 1 CM, then
share 1 CM, then  or
or  for some constants
for some constants  and
and  that satisfy
that satisfy  and
and  .
.
In this paper, we improve Theorems B and C to meromorphic functions and obtain the following results.
Theorem 1.10.
Let  and
and  be transcendental meromorphic functions with finite order. Suppose that
be transcendental meromorphic functions with finite order. Suppose that  is a nonzero constant and
is a nonzero constant and  . If
. If  ,
,  and
and  share 1 CM, then
share 1 CM, then  or
or  , where
, where  .
.
Theorem 1.11.
Under the conditions of Theorem 1.10, if  ,
,  and
and  share 1 IM, then
share 1 IM, then  or
or  , where
, where  .
.
Remark 1.12.
Let  and
and  ,
,  . Thus,
. Thus,  and
and  share the value 1 CM. From above, the case
share the value 1 CM. From above, the case  , where
, where  may occur in Theorems 1.10 and 1.11.
may occur in Theorems 1.10 and 1.11.
From the proof of Theorem 1.11 and (2.7) below, we obtain easily the next result.
Corollary 1.13.
Let  and
and  be transcendental entire functions with finite order, and
be transcendental entire functions with finite order, and  be a nonzero complex constant. If
be a nonzero complex constant. If  ,
,  and
and  share 1 IM, then
share 1 IM, then  or
or  , where
, where  .
.
2. Some Lemmas
The difference logarithmic derivative lemma of functions with finite order, given by Chiang and Feng [20, Corollary 2.5], Halburd and Korhonen [21, Theorem 2.1], plays an important part in considering the difference Nevanlinna theory. Here, we state the following version.
Lemma 2.1 (see [22, Theorem 5.6]).
Let  be a transcendental meromorphic function of finite order, and let
be a transcendental meromorphic function of finite order, and let  . Then
. Then

for all  outside of a set of finite logarithmic measure.
outside of a set of finite logarithmic measure.
Lemma 2.2 (see [20, Theorem 2.1]).
Let  be a transcendental meromorphic function of finite order. Then,
be a transcendental meromorphic function of finite order. Then,

For the proof of Theorem 1.4, we need the following lemma.
Lemma 2.3.
Let  be a transcendental meromorphic function of finite order. Then,
be a transcendental meromorphic function of finite order. Then,

Proof.
Assume that  , then
, then

Using the first and second main theorems of Nevanlinna theory and Lemma 2.1, we get

thus, we get the (2.3).
In order to prove Theorem 1.5 and Corollary 1.13, we also need the next result.
Lemma 2.4.
Let  be a transcendental meromorphic function with finite order,
be a transcendental meromorphic function with finite order,  . Then
. Then

If  is a transcendental entire function with finite order, and
is a transcendental entire function with finite order, and  ,
,  , then
, then

Proof.
We deduce from Lemma 2.1 and the standard Valiron-Mohon'ko [23] theorem,

Thus, (2.6) follows from (2.8). If  is entire and
is entire and  ,
,  , then from above, we get
, then from above, we get

Moreover,  follows by Lemma 2.2. Thus (2.7) is proved.
follows by Lemma 2.2. Thus (2.7) is proved.
Lemma 2.5 (see [17, Lemma 3]).
Let  and
and  be two nonconstant meromorphic functions. If
be two nonconstant meromorphic functions. If  and
and  share 1 CM, then one of the following three cases holds:
share 1 CM, then one of the following three cases holds:
-
(i)
 ,
, -
(ii)
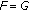 ,
, -
(iii)
 ,
,
where  denotes the counting function of zeros of
denotes the counting function of zeros of  such that simple zeros are counted once and multiple zeros are counted twice.
such that simple zeros are counted once and multiple zeros are counted twice.
For the proof of Theorem 1.11, we need the following lemma.
Lemma 2.6 (see [16, Lemma 2.3]).
Let  and
and  be two nonconstant meromorphic functions, and
be two nonconstant meromorphic functions, and  and
and  share 1 IM. Let
share 1 IM. Let

If  , then
, then

3. Proof of the Theorems
Proof of Theorem 1.2.
Since  is a transcendental meromorphic function, assume that
is a transcendental meromorphic function, assume that  , then we can get
, then we can get

Using the second main theorem, we have

So the condition  implies that
implies that  must have infinitely many zeros.
must have infinitely many zeros.
Proof of Theorem 1.7.
Let

We proceed to prove that  has infinitely many zeros, which implies that
has infinitely many zeros, which implies that  has infinitely many zeros. We first prove that
has infinitely many zeros. We first prove that

Applying the first main theorem and Lemma 2.2, we observe that

From (3.5), we easily obtain the inequality (3.4). Concerning the zeros and poles of  , we have
, we have


Using the second main theorem, Lemma 2.2, (3.6) and (3.7), we get

Since  , then (3.8) implies that
, then (3.8) implies that  has infinitely many zeros, completing the proof.
has infinitely many zeros, completing the proof.
Remark 3.1.
It is easy to know that if  , then (3.7) can be replaced by
, then (3.7) can be replaced by

which implies that  in Theorem 1.7.
in Theorem 1.7.
Proof of Theorem 1.10.
Let  and
and  . Thus,
. Thus,  and
and  share the value 1 CM. Suppose first that
share the value 1 CM. Suppose first that  and
and  . From the beginning of the proof of Theorem 1.2, we obtain
. From the beginning of the proof of Theorem 1.2, we obtain

Moreover, from Lemma 2.2, it is easy to get

Using the second main theorem, we have

Thus,

Similarly, we obtain

Therefore, from (3.13) and (3.14),  follows. From the definition of
follows. From the definition of  , we get
, we get

Similarly, we can get



Thus,

Then, from (3.10), and (3.19), we have

which is in contradiction with  .Therefore, applying Lemma 2.5, we must have either
.Therefore, applying Lemma 2.5, we must have either  or
or  . If
. If  , thus,
, thus,  . Let
. Let  . Assume that
. Assume that  is not a constant. Then we get
is not a constant. Then we get

Thus, from Lemma 2.2, we get

which is a contradiction with  . Hence
. Hence  must be a constant, which implies that
must be a constant, which implies that  , thus,
, thus,  and
and  .
.
If  , implies that
, implies that

Let  , similar as above,
, similar as above,  must be a constant. Thus
must be a constant. Thus  ,
,  follows; we have completed the proof.
follows; we have completed the proof.
Proof of Theorem 1.11.
Let  and
and  , let
, let  be defined in Lemma 2.6. Using the similar proof as the proof of Theorem 1.10 up to (3.18), combining with Lemma 2.6 and
be defined in Lemma 2.6. Using the similar proof as the proof of Theorem 1.10 up to (3.18), combining with Lemma 2.6 and

we can get

which is in contradiction with  . Thus, we get
. Thus, we get  . The following is standard. For the convenience of reader, we give a complete proof here. By integratiing (2.10) twice, we have
. The following is standard. For the convenience of reader, we give a complete proof here. By integratiing (2.10) twice, we have

which implies  . From (3.10)-(3.11), thus,
. From (3.10)-(3.11), thus,


In the following, we will prove that  or
or  .
.
Case 1 ( ).
).
If  , then by (3.26), we get
, then by (3.26), we get

Combining the Nevanlinna second main theorem with Lemma 2.4 and (3.27), we have

This implies  , which is in contradiction with
, which is in contradiction with  . Thus,
. Thus,  , hence
, hence

Using the same method as above,

which is also a contradiction.
Case 2 ( ,
,  ).
).
From (3.26), we have

Similarly, we also can get a contradiction. Thus,  follows, implies that
follows, implies that  . Thus, we get
. Thus, we get  and
and  .
.
Case 3 ( ,
,  ).
).
From (3.26), we obtain

Similarly, we get a contradiction,  follows. Thus, we get
follows. Thus, we get  also implies
also implies  ,
,  . Thus, we have completed the proof.
. Thus, we have completed the proof.
References
Hayman WK: Meromorphic Functions, Oxford Mathematical Monographs. Clarendon Press, Oxford, UK; 1964:xiv+191.
Laine I: Nevanlinna Theory and Complex Differential Equations, de Gruyter Studies in Mathematics. Volume 15. Walter de Gruyter & Co., Berlin, Germany; 1993:viii+341.
Yang C-C, Yi H-X: Uniqueness Theory of Meromorphic Functions, Mathematics and Its Applications. Volume 557. Kluwer Academic Publishers, Dordrecht, The Netherlands; 2003:viii+569.
Hayman WK: Research Problems in Function Theory. The Athlone Press, London, UK; 1967:vii+56.
Hayman WK: Picard values of meromorphic functions and their derivatives. Annals of Mathematics 1959, 70: 9-42. 10.2307/1969890
Mues E: Über ein Problem von Hayman. Mathematische Zeitschrift 1979,164(3):239-259. 10.1007/BF01182271
Bergweiler W, Eremenko A: On the singularities of the inverse to a meromorphic function of finite order. Revista Matemática Iberoamericana 1995,11(2):355-373.
Laine I, Yang C-C: Value distribution of difference polynomials. Proceedings of the Japan Academy. Series A 2007,83(8):148-151. 10.3792/pjaa.83.148
Liu K, Yang L-Z: Value distribution of the difference operator. Archiv der Mathematik 2009,92(3):270-278. 10.1007/s00013-009-2895-x
Liu K: Value distribution of differences of meromorphic functions. Rocky Mountain Journal of Mathematics. In press
Liu K, Laine I: A note on value distribution of difference polynomials. Bulletin of the Australian Mathematical Society 2010,81(3):353-360. 10.1017/S000497270900118X
Zhang J: Value distribution and shared sets of differences of meromorphic functions. Journal of Mathematical Analysis and Applications 2010,367(2):401-408. 10.1016/j.jmaa.2010.01.038
Liu K: Zeros of difference polynomials of meromorphic functions. Results in Mathematics 2010,57(3-4):365-376. 10.1007/s00025-010-0034-4
Fang ML, Hua XH: Entire functions that share one value. Nanjing Daxue Xuebao 1996,13(1):44-48.
Qiu HL, Fang ML: On the uniqueness of entire functions. Bulletin of the Korean Mathematical Society 2004,41(1):109-116.
Xu JF, Yi HX: Uniqueness of entire functions and differential polynomials. Bulletin of the Korean Mathematical Society 2007,44(4):623-629. 10.4134/BKMS.2007.44.4.623
Yang C-C, Hua XH: Uniqueness and value-sharing of meromorphic functions. Academiæ Scientiarum Fennicæ 1997,22(2):395-406.
Lahiri I, Pal R: Non-linear differential polynomials sharing 1-points. Bulletin of the Korean Mathematical Society 2006,43(1):161-168.
Qi X-G, Yang L-Z, Liu K: Uniqueness and periodicity of meromorphic functions concerning the difference operator. Computers & Mathematics with Applications 2010,60(6):1739-1746. 10.1016/j.camwa.2010.07.004
Chiang Y-M, Feng S-J:On the Nevanlinna characteristic of
 and difference equations in the complex plane. Ramanujan Journal 2008,16(1):105-129. 10.1007/s11139-007-9101-1
and difference equations in the complex plane. Ramanujan Journal 2008,16(1):105-129. 10.1007/s11139-007-9101-1Halburd RG, Korhonen RJ: Difference analogue of the lemma on the logarithmic derivative with applications to difference equations. Journal of Mathematical Analysis and Applications 2006,314(2):477-487. 10.1016/j.jmaa.2005.04.010
Halburd RG, Korhonen RJ: Meromorphic solutions of difference equations, integrability and the discrete Painlevé equations. Journal of Physics. A 2007,40(6):R1-R38. 10.1088/1751-8113/40/6/R01
Mohon'ho AZ: The Nevanlinna characteristics of certain meromorphic functions. Teoriya Funktsiu i, Funktional'nyu i Analiz i ikh Prilozheniya 1971, (14):83-87.
Acknowledgments
The authors thank the referee for his/her valuable suggestions to improve the present paper. This work was partially supported by the NNSF (no. 11026110), the NSF of Jiangxi (nos. 2010GQS0144 and 2010GQS0139) and the YFED of Jiangxi (nos. GJJ11043 and GJJ10050) of China.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Liu, K., Liu, X. & Cao, T. Value Distributions and Uniqueness of Difference Polynomials. Adv Differ Equ 2011, 234215 (2011). https://doi.org/10.1155/2011/234215
Received:
Accepted:
Published:
DOI: https://doi.org/10.1155/2011/234215
 ,
,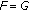 ,
, ,
, and difference equations in the complex plane. Ramanujan Journal 2008,16(1):105-129. 10.1007/s11139-007-9101-1
and difference equations in the complex plane. Ramanujan Journal 2008,16(1):105-129. 10.1007/s11139-007-9101-1